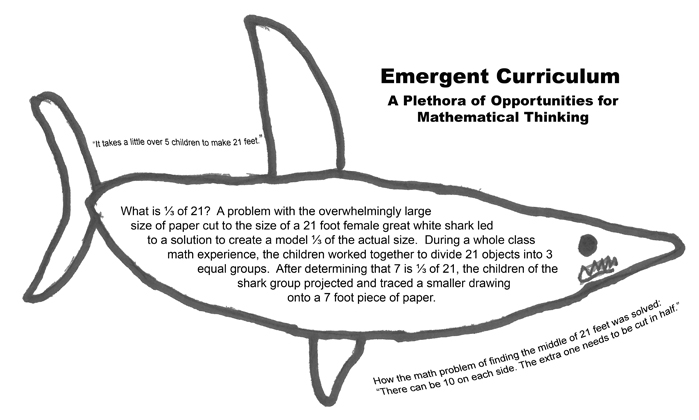
Children explore problem solving, cause and effect, and time-space relationships through concrete, active experiences. They learn the principles of math through the use of objects first (including their own bodies) then graphic representation, and ultimately symbolic systems such as number and numeral. The materials with which the children explore may be real concrete objects but the relationship of one object to another is an abstract concept. This needs to be internalized by the child through many direct experiences and with the problem-solving challenges offered by teachers.
With a solid foundation of direct experience, children move naturally into more complex, abstract procedures. Counting objects (as opposed to rote sequencing of number), one-to-one correspondence, manipulation of equal and unequal sets, graphing relationships, measurement, spatial form and boundaries, classifying, ordering, perceiving and creating patterns are all part of the logical mathematical curriculum. These key elements may be focused upon as separate activities, but they are also integrated into everyday activities at our school so that children learn that these mathematical and logical processes are useful tools in daily life. As children mature, they begin to write and document these experiences. They learn to represent relationships using numerical symbol systems and they translate data into coded graphs. They learn to apply their skills as tools for problem solving.